You’ve learned about momentum for singular objects. The question now, is what happens if you have more than one object? How can momentum be used to understand what happens when two objects collide? The answer comes from a law known as the law of the conservation of momentum. In this post, we’ll cover the law of conservation of momentum, the difference between elastic and inelastic collisions, and examples of each.
What We Review
What Is Conservation Of Momentum?
Momentum is, effectively, a measure of how hard it will be to stop a moving object. This is a common value in physics as it applies to every moving object. We already learned how to calculate momentum so now we’ll learn how actions affect momentum.
Definition Of The Law Of Conservation Of Momentum
| The Law of Conservation of Momentum The law of conservation of momentum states that all of the momentum in a system before an action takes place must be equal to all of the momentum in the system after the action. |
In summary, for all actions, momentum must be conserved. This is true when looking at the movement of a single object, or interactions between two or more objects. This is also true for linear momentum and angular momentum.
Momentum is the product of an object’s mass and speed. You may be able to see a link to kinetic energy with those values. Although momentum and energy are not equivalent, the same principles that apply to the conservation of energy also apply to the conservation of momentum. If you have some amount of something, that thing cannot vanish because of an action. It can be transferred from one object to another, but it cannot vanish.
Applications Of The Conservation Of Momentum
The conservation of momentum appears in many areas of physics. It helps engineers better understand what happens during car crashes to help keep us safe. In sports, it explains what will happen when hitting a baseball or tackling another player. These would mostly be cases of linear momentum, but this law applies to angular momentum as well. We’ll be sticking with linear momentum for the rest of this post, but know that this same idea can help predict how objects move when in orbit or when changing direction.

Elastic And Inelastic Collisions
We often utilize the law of conservation of momentum when looking at collisions. This law applies to both elastic and inelastic collisions.

An elastic collision is one in which two objects collide and then bounce apart. This can be a basketball bouncing off the floor or one ball in a game of pool bouncing off another. An inelastic collision is the opposite of an elastic collision. In these, the two objects collide and then stick together. This can be a car crash or a cooked piece of pasta sticking to a wall.
Similarities And Differences Between Elastic And Inelastic Collisions
The main similarity in both of these collisions is that momentum is conserved. In reality, this usually has to account for energy lost to the production of sound or heat. For the sake of your physics courses, though, you genuinely won’t account for these things. The main difference between elastic and inelastic collisions is that elastic collisions bounce apart while inelastic collisions stick together.
If you have trouble figuring out how to know if a collision is elastic or inelastic, it’s important to try to picture what’s actually happening. With words like “bounce”, “moved apart after colliding”, or “separated”, you’ve got an elastic collision. On the other hand, if you have “stuck together”, “moved together after colliding”, or “becomes embedded”, then it’s an inelastic collision.
| Elastic Collision | Inelastic Collision | |
| Objects Bounce Apart | ||
| Objects Stick Together | ||
| Momentum is Conserved |
The Conservation Of Momentum Equation
The conservation of momentum equation is a relatively straightforward one:
| Conservation of Momentum Equation \Sigma p_{i}=\Sigma p_{f} \Sigma m_{i}v_{i}=\Sigma m_{f}v_{f} |
The reason we use the \Sigma symbol is to show that this is true for several momentum values. You could also substitute in the momentum equation and write the equation for the conservation of momentum using masses and velocities.
Here, we’ve used i to indicate initial values and f final values respectively. We often use numbers to represent these different values, but you’ll see why we used letters here in a moment.
How To Use The Conservation Of Momentum Equation
You’ve probably seen the conservation of momentum mentioned with collisions. This law can be applied to elastic or inelastic collisions by expanding our equation a bit to include two objects:
m_{1i}v{1i}+m_{2i}v_{2i}=m_{1f}v_{1f}+m_{2f}v_{2f}
We can use numbers to note whether we are talking about object one or two and letters to note whether we are talking about the value before (i for “initial”) or after (f for “final”) an action has taken place. This can help us predict how an object will behave after a collision because we can see how momentum needs to be balanced.
If before the collision, object one had very little momentum while object two had a lot, and then after the collision, object one now has a lot of momentum, we can predict that object two should have less momentum after the collision. The more you work with the concept, the better you will get at making predictions around changes in mass or velocity after collisions.
Conservation Of Momentum In The Lab
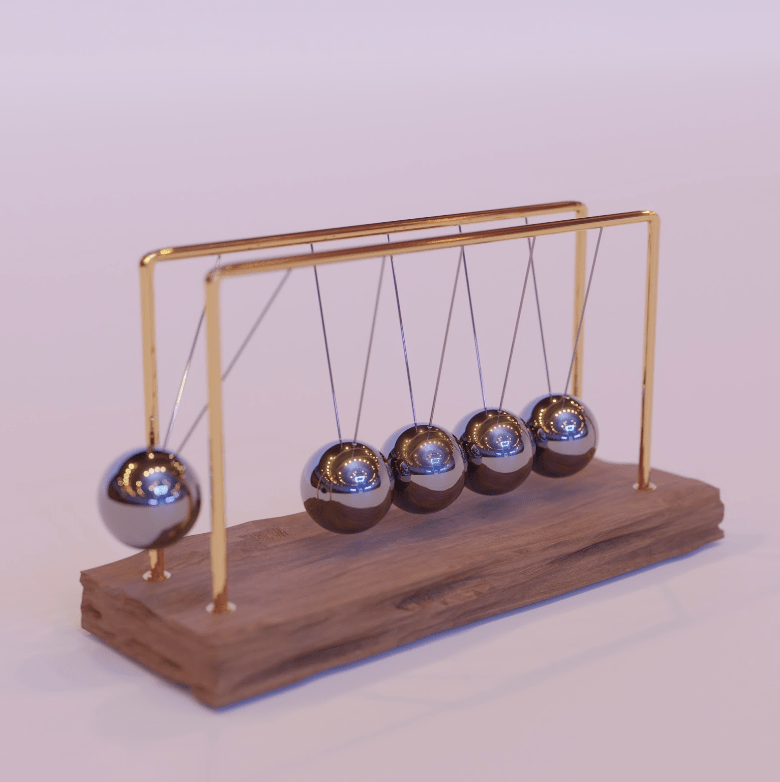
There are many examples of the conservation of momentum in the real world. A famous one is Newton’s cradle, where momentum is transferred from one ball through several others to finally launch the ball on the opposite end. The Moon’s motion is an excellent example of the conservation of angular momentum if you’d like to learn more about it. For now, let’s look at an in-lab experiment where this can be proven.
A Classic Experiment
To run this experiment, you’ll need two carts, a frictionless air track, and a way to measure velocity. You can also utilize an online simulator. To start, we’ll model an elastic collision and then an inelastic collision.
Modeling an Elastic Collision
To do this, we’ll record the mass of our two carts before pushing them toward each other. You’ll need to measure the initial and final velocities each time you do this.
| Initial Velocity of Cart 1 (m_{1i}= 1\text{ kg}) v_{1i} | Initial Velocity of Cart 2 ( m_{2i}= 3\text{ kg}) v_{2i} | Final Velocity of Cart 1 (m_{1i}= 1\text{ kg}) v_{1f} | Final Velocity of Cart 2 ( m_{2i}= 3\text{ kg}) v_{2f} | |
| Trial #1 | 5\text{ m/s} | -0.9\text{ m/s} | -4\text{ m/s} | 2\text{ m/s} |
| Trial #2 | 5.2\text{ m/s} | -1\text{ m/s} | -4.2\text{ m/s} | 2.2\text{ m/s} |
| Trial #3 | 4.9\text{ m/s} | -0.9\text{ m/s} | -4.1\text{ m/s} | 2\text{ m/s} |
Now, we can use this data to check that the conservation of momentum equation works. To do this, we simply need to plug in our values and see if the equation is balanced. Keep in mind that your data likely has some degree of error so these values may not work out perfectly each time. So, we’ll set up a table to see how similar the two sides are. We’ll show our work for the first trial, but after that, we’ll just show the results.
| m_{1i}v_{1i}+m_{2i}v_{2i} | m_{1f}v_{1f}+m_{2f}v_{2f} | |
| Trial #1 | 1\text{ kg}\cdot 5\text{ m/s}+3\text{ kg}\cdot -0.9\text{ m/s} =5\text{ kg m/s}-2.7\text{ kg m/s}=2.3\text{ kg m/s} | 1\text{ kg}\cdot -4\text{ m/s}+3\text{ kg}\cdot 2\text{ m/s} =-4\text{ kg m/s}+6\text{ kg m/s} =2\text{ kg m/s} |
| Trial #2 | 2.2\text{ kg m/s} | 2.4\text{ kg m/s} |
| Trial #3 | 2.2\text{ kg m/s} | 2\text{ kg m/s} |
As expected, not all of these values are a perfect match. They are close enough, however, that the differences could be due to human error or imprecise measurements. For this reason, we can conclude that our experiment would prove the law of the conservation of momentum holds for elastic collisions. Now let’s check if this is true for inelastic collisions.
Modeling an Inelastic Collision
Now, we’ll repeat the experiment for inelastic collisions. This time, we will make sure the two carts stick together after colliding. We will then record our data and check that the equation holds in the same way. One common way to make the two carts stick together is to add velcro to the ends of the carts.
Note: Because the carts stick together after, we will only have one mass and one velocity value on the right-hand side of our equation. This means our table and notation will look a little bit different.
| Initial Velocity of Cart 1 (m_{1i}= 1\text{ kg}) v_{1i} | Initial Velocity of Cart 2 ( m_{2i}= 3\text{ kg}) v_{2i} | Combined Velocity of Both Carts(m_{f}= 4\text{ kg}) v_f | |
| Trial #1 | 4.8\text{ m/s} | 0\text{ m/s} | 1.2\text{ m/s} |
| Trial #2 | 5.3\text{ m/s} | 0\text{ m/s} | 1.3\text{ m/s} |
| Trial #3 | 5.1\text{ m/s} | 0\text{ m/s} | 1.2\text{ m/s} |
Now that we have our data, we can set up the same table we’d had before to check the equation for the conservation of momentum. Again, we’ll show work for the first trial but none of the others.
| m_{1i}v{1i}+m_{2i}v_{2i} | (m_{1f}+m_{2f})v_{f} | |
| Trial #1 | 1\text{ kg}\cdot 4.8\text{ m/s}+3\text{ kg}\cdot 0\text{ m/s}=4.8\text{ kg m/s}+0\text{ kg m/s}=4.8\text{ kg m/s} | (1\text{ kg}+3\text{ kg})\cdot 1.2\text{ m/s}=4.8\text{ kg m/s} |
| Trial #2 | 5.3\text{ kg m/s} | 5.2\text{ kg m/s} |
| Trial #3 | 5.1\text{ kg m/s} | 4.8\text{ kg m/s} |
Again, not all of our numbers match perfectly, but they are close enough to conclude the conservation of momentum applies to inelastic collisions.
Conclusion
Now we’ve learned about the conservation of momentum, the difference between elastic and inelastic collisions, and how to apply the conservation of momentum to different types of collisions. This is one of the fundamental laws that help shape the universe and you’ll continue to see it throughout your physics journey.