Hi, guys! Welcome to another of our Ultimate AP® Chemistry Guide series! This time, we’re going to teach you all about electrochemistry. We’ll start off with a quick overview of what electrochemical reactions are. Then we’ll go over the terms “oxidation” and “reduction”, what redox reactions are, and how to balance them. Once you can define those terms without even thinking twice, you will learn how electrochemical reactions can be described in terms of free energy change and how those calculations tie in with other thermodynamic equations. We will then outline the conditions under which a voltaic cell is created, and how it functions. Finally, we will briefly cover the topic of corrosion, and how metals can be protected from it.
Quick Navigation
7. Free Energy
8. Standard Reduction Potential
10. Corrosion
Let’s go back to basics for a second.
Question: What is a chemical reaction?
Answer: A chemical reaction occurs when two substances (reactants) interact with each other and undergo a chemical transformation to become other substances, generally with different properties than they had before (products). Chemical reactions can be described, among other things, in terms of their stoichiometry and of their free energy change (\Delta G^\circ ). So, too, can electrochemical reactions. In much the same way as chemical reactions, electrochemical reactions are defined in terms of products being made from reactants. The main difference between a standard chemical reaction and an electrochemical reaction is (no surprise): electrons (e^-).
Electrochemistry – Essential Knowledge 3.C.3
Electrochemical reactions, as the name implies, classify chemical reactions that involve the flow of electricity. In other words, they involve the movement of electrons from one substance to another. This movement of electrons is more commonly referred to as an electrical current. Current is measured in units of Amperes (\text{amp}), where one amp of current is the movement of one coulomb of electric charge per second (\text{A = C s}^{-1}). The flow of electrons can be described as a pair of corresponding reactions: oxidation and reduction.
The term oxidation state is a measure of the number of electrons relative to the number of protons in an atom. An atom in the “ground state” has an equal number of electrons and protons, and therefore, we say it has an oxidation number of 0. The term oxidation state can be confusing because the first thing you think about is the word “oxygen.” This nomenclature is not a coincidence.
The term “oxidation” was coined by French Chemist Antoine Lavoisier (1743-1794), who discovered chemical reactions which caused molecules to bind to oxygen, or, become oxidized. Many years later, other chemists figured out that when atoms bonded together in a molecule through covalent bonds, the more electronegative atom “grabs” more of the electrons, and they quantified this relationship as a change in the oxidation state of an atom. Let’s use an example.

Redox Reactions – Essential Knowledge 3.C.3, 6.A.1
Rusting is a type of redox reaction where solid iron reacts with molecular oxygen to form Iron (III) Oxide. When the iron becomes oxidized (i.e., when oxygen adds to it) the oxidation state of iron changes from the ground state 0 to +3. So as we see, when compounds become oxidized, their oxidation number increases. Another way to think about the oxidation state is that in this reaction, oxygen steals some of the electrons off of iron to become more stable, and therefore, it “gains” positive charge. On the other hand, when molecular oxygen reacts with Fe\left( s \right), its oxidation state changes from the ground state 0 to -2, so the oxidation state of the reduced molecule becomes more negative. In other words, oxygen gains electrons in this reaction and acquires a net negative charge. These types of reactions are known as oxidation-reduction reactions, or redox.
While a Redox Reactions is technically one single reaction, it can be split into a pair of concurrent reactions. When added together, the oxidation and reduction half-reactions add up to a complete chemical reaction. When writing out half-reactions, we include the number of electrons being moved to help us see the flow of electrons in the reaction. But where do the electrons go? Well, in the same way that the number of atoms in a reaction have to balance across the reaction using stoichiometry, the charge of all the species have to balance across the reaction. Let’s use the rusting of iron to explain this concept.
First let’s write the balanced stoichiometric reaction of rusting iron, considering that the final product is F{ e }_{ 2 }{ O }_{ 3\left( s \right) }:
F{ e }_{ (s) } +{O }_{ 2\left( g \right)} = F{ e }_{ 2 }{ O }_{ 3\left( s \right) }
4F{ e }_{ (s) }{ +3O }_{ 2\left( g \right) }=2F{ e }_{ 2 }{ O }_{ 3\left( s \right) }
Now, let’s divide this equation into its oxidation and reduction portions. How do we know what number to assign the oxidation state of each atom? Well, elements in their ground state have a defined oxidation state of zero. As far as the products, we know what for the charges to be balanced, the net electrical charge on both sides must be equal. So, if the sum of the charges on the left is zero, the sum of charges on the right must be zero as well.
Electronegativity – Essential Knowledge 2.C.1.
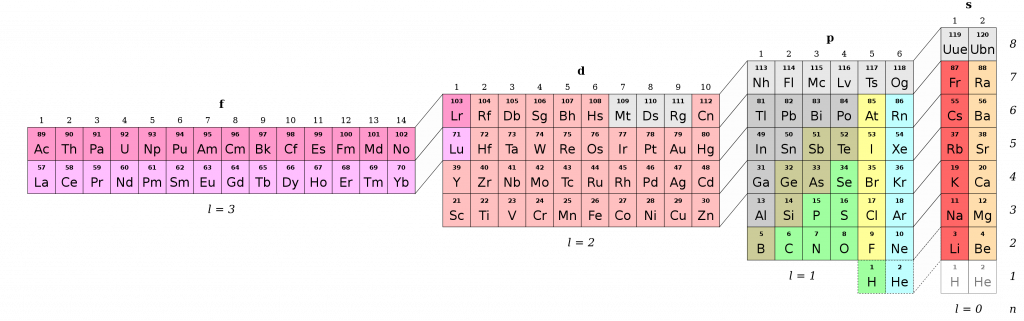
Now to know what oxidation state to assign oxygen and iron, you have to consider the electronegativities of the atoms involved. Let’s remind us of the trend in periodic behavior:

The most electronegative atom is fluorine (Z = 7, top right), and the least electronegative atom is francium (Z = 87, bottom left). The oxidation state of a molecule directly relates to the manner in which the covalent bond is shared between bonded atoms. The more electronegative atom attracts the electrons more strongly to its nucleus because of the added stability this arrangement gives to the molecule (electron configuration reminder: a full shell is more stable than a partially-filled shell). Therefore, the electron spends more time revolving around the more electronegative atom. We designate this relationship by assigning the most electronegative atom a negative oxidation state,= to indicate that the electrons “belong” to it, whereas we show the less electronegative atom has “lost” electrons and therefore has a positive oxidation state.
Electron Configuration – Essential Knowledge 1.B.2
But where do the electrons come from? And where do they go? Well, as we know, chemicals are all fundamentally made up of atoms. Atoms, in turn, are classified by the number of protons in their nucleus, which determines the total positive charge of each element. And when the atom has an equal number of electrons orbiting the nucleus, the atom has a net neutral charge. However, the stability of an element is not determined by whether or not it has a neutral charge. In fact, the stability of an element has more to do with its electron configuration.
Atoms, like glasses, do not like being half-empty or half-full. Rather, they prefer having an electron configuration which results in them having either full or empty orbitals. Determining which orbitals electrons inhabit is done by “building up” the atom’s valence electrons (Aufbau principle). In most cases, however, the most stable version of an element is not one where the number of electrons equals the number of protons. Rather, it is energetically favorable for elements to either gain or lose electrons to become more stable.
Going back to our balanced stoichiometric reaction, 4F{ e }_{ (s) }{ +3O }_{ 2\left( g \right) }=2F{ e }_{ 2 }{ O }_{ 3\left( s \right) }, we will first assign oxidation states to all the elements, and then write out the individual half-reactions it is composed of (oxidation of iron and reduction of oxygen). You will also learn some general tips about how to assign oxidation numbers. Are you ready? Let’s hit it!
Oxidation States – Essential Knowledge 3.B.3
Assigning oxidation number can be a big issue for many students because there are millions of chemical compounds each with their own identities. The information overload can cause you to panic, especially during the AP® exam when it is more crucial for you to stay calm. So we’re going to teach you a great method to remember how to assign oxidation states that you’ll be able to use on the AP® exam. It involves using the periodic table, which you get for free!

As we previously discussed, oxidation state relates to electronegativity, and it also relates to electron configuration. Therefore, think about the periodic table when we go through these rules, and memorize them in terms of the table. When you finish applying these rules, assign the remaining oxidation states in the compound by keeping in mind that the sum of all oxidation state numbers must equal the net charge on the molecule.
# |
Chemical Species |
Oxidation State |
Exceptions |
| 1 | Elemental Form | 0 | |
| 2 | Monoatomic Ions | Ionic Charge | |
| 3 | Group 1A, 2A Metals | +1, +2 | |
| 4 | Oxygen | -2 | Except in peroxides and F_2O |
| 5 | Hydrogen | +1 | Except in metal hydrides where it is -1 |
| 6 | Fluorine | -1 | |
| 7 | Chlorine, Bromine, Iodine | –1 | Except in compounds with O or F |
| 8 | Groups 7A, 6A, 5A | -1, -2, -3 | *in binary compounds with metals |
So what do we mean when we say “keep the periodic table in mind”? Let’s take a look at Rule 3: “Group 1A, 2A Metals – +1, +2“. The reason for this rule is that alkali and alkaline earth metals are much more stable without any electrons in their outlying s-orbital, and they tend to lose those electrons to form more stable ions. Looking at the periodic table shows you this relationship visually. In the ground state, those metals will have outlying electrons, which is energetically unfavorable. The same line of reasons can be used for Rules 6 & 8, where we can see how the position of these elements can affect the number of electrons they lose to become stable.
Rule 7 makes sense when considering the difference in electronegativities between the halogens (Group 7A). Electronegativity decreases as you go down a group, and the most electron negative atoms are assigned the negative oxidation state. Since fluorine is the most electronegative element, followed by oxygen, any compounds formed between fluorine/oxygen and another halogen will cause a positive oxidation state to be placed on the halogen. In every other case, however, the halogens will be the most electronegative atoms, so they will have an oxidation state of -1.
So, apply the rules, then assign the remaining oxidation states based on “the sum of oxidation states equals the net charge”. Let’s practice assigning oxidation numbers to our rusting iron example. The balanced chemical reaction is:
4F{ e }_{ (s) }{ +3O }_{ 2\left( g \right) }=2F{ e }_{ 2 }{ O }_{ 3\left( s \right) }
Rule 1: F{ e }_{ (s) } and { O }_{ 2\left( g \right) } have an oxidation state of zero.
Rule 4: Oxygen’s oxidation state is -2.
To get the oxidation state of Fe, get the total number of atoms of each element in the compound and balance it to equal the net charge on the compound.
4Fe+6{ O }^{ 2- }= 0
4Fe+\left( -12 \right) = 0
4Fe= +12
Fe=+3
We’re done! You now possess all the knowledge necessary to assign oxidation states correctly. Armed with this knowledge, we will now balance the redox reaction of iron rusting and generalize that example to balancing redox equations in general.
Balancing Redox Equations – Essential Knowledge 3.B.3, 3.C.3, 6.A.1
Since we now know the oxidation states of the products and reactants, we can split the balanced reaction up into its constituent oxidation and reduction reactions. You designate the oxidation state by writing it as if it were an electric charge on the element. The balanced oxidation half-reaction would look like this:
4F{ e }_{ \left( s \right) }=4F{ e }^{ 3+ } \rightarrow F{ e }_{ \left( s \right) }=F{ e }^{ 3+ }
And the balanced reduction half-reaction would look like this:
3{ O }_{ 2\left( g \right) }=6{ O }^{ 2- }\rightarrow { O }_{ 2\left( g \right) }=2{ O }^{ 2- }
The same rules for balancing the electric charge across the reaction apply to half reactions as well. Once oxidized, iron has an oxidation state of 3+. We indicate that the iron atom loses three electrons to balance this positive charge. This is written as:
F{ e }_{ \left( s \right) }=F{ e }^{ 3+ }+3{ e }^{ - }
Now the net charge on both sides of the equation is zero, and the reaction is balanced. The reduction half-reaction would look like this once accounting for the electrons:
4{ e }^{ - }+{ O }_{ 2\left( g \right) }=2{ O }^{ 2- }
Notice that we wrote the electrons in on the reactant side of the equation, in this case, to balance it out because it was the products that had the charge on them. This is generally the case for reduction half-reactions.

We now have the two balanced half-reactions rusting. What do we do with this information? Well, we now add the two reactions together and balance that! The goal here is to multiply each half-reaction by the least common denominator of the electrons on each side so that when we add the reactions together, the electrons cancel out.
4 \times Fe_{(s)} = (Fe^{3+} + 3e^-) \times 4
3 \times (4e^- + O_{2(g)}) = 2O^{2-} \times 3
4Fe_{(s)} = 4Fe^{3+} + 12e^-
12e^- + 3O_{2(g)} = 6O^{2-}
4Fe_{(s)} + 3O_{2(g)} = 4Fe^{3+} + 6O^{2-} = 2Fe_2O_3
The final product of this reaction, F{ e }_{ 2 }{ O }_{ 3 }, or Iron (III) Oxide, is rust. There are other electrochemical reactions that, besides electrons, also produce protons (H^+), which you also have to balance until they cancel out. We will see examples of that later. In the meantime, let’s look at the process one more time step-by-step to make sure we got it right.
1) Balance the chemical reaction.
2) Assign oxidation states to all elements.
3) Write out the two redox half reactions.
4) Balance the charge on each half-reaction by adding electrons.
5) Add the two half reaction together to arrive at your final balanced electrochemical reaction.
Free Energy – Essential Knowledge 5.E.3, 5.E.4, 6.D.1
We have finished learning about how to classify an electrochemical reaction stoichiometrically. Now we will find out how to classify it in thermodynamic terms (namely, how the electrical component can be incorporated into discussions of free energy change \Delta G^\circ ). Free energy is a measure of the potential energy of a molecule. When substances go from reactants to products, they undergo a free energy change called noted as \Delta G^\circ .
Here is the main equation relating to electrochemistry that will be provided to you on the AP® Chemistry Exam Equation Sheet:
\Delta { G }^{\circ }=-nF{ E }^{\circ }_{ cell }
…where F = \text{Faraday’s Constant} = 96{,}485 \text{ Coulombs/mol e}^{-}, { E }^{\circ }_{cell} = standard reduction potential, and n = number of moles of electrons.
The { E }_{ cell }^{\circ }, or standard reduction potential, is an experimentally-determined value of the electrical potential in volts (V) across the two half-reactions. The standard reduction potential for the hydrolysis of hydrogen gas into protons and electrons is defined as 0. Since n must be a positive integer and F is a constant, any values of { E }^{\circ }_{ cell } that are positive will give a negative \Delta { G }^{\circ }, so the reaction is spontaneous in the direction it is written. On the other hand, if { E }_{ cell }^{\circ } is negative, then \Delta { G }^{\circ } is positive, and the reaction as written becomes unfavorable and non-spontaneous.

Although we won’t get into it, keep this diagram in mind when trying to figure out the relationship between free energy, standard reduction potential, and the equilibrium concentration of products and reactants in a reaction. The bolded equation in red will be discussed next up!
Standard Reduction Potential – Essential Knowledge 3.C.3
Let’s remind us what a positive voltage means with this diagram below:

As you see, having a positive value for { E }^{\circ }_{ cell } means that the electrons are flowing in the opposite direction. It’s important to remember that { E }^{\circ }_{ cell } measures the potential across the reactions going from right to left. Half-reactions with a negative { E }^{\circ }_{ cell } value are spontaneous in the direction they are written since a negative value of { E }^{\circ }_{ cell } results in a negative \Delta { G }^{\circ }. This is a common pitfall for many AP® Chem students: The flow of electrons is opposite that of the movement of charge, and the voltage measures the flow of charge.
Standard Reduction Potential |
|||
Oxidant |
\rightleftharpoons |
Reductant |
{ E }^{\circ }(\text{V}) |
| 2 Cl^- | \rightleftharpoons | Cl_{2(g)} + 2 e^- | -1.36 |
| Ag_{(s)} | \rightleftharpoons | Ag^+ +e^- | -0.7996 |
| 4 OH^-_{(aq)} | \rightleftharpoons | O_{2(g)}+ 2 H_2O + 4e^- | -0.401 |
| Cu_{(s)} | \rightleftharpoons | Cu^{2+} + 2e^- | -0.337 |
| Ag_{(s)} + Cl^- | \rightleftharpoons | AgCl_{(s)} +e^- | -0.22233 |
| H_{2 (g)} | \rightleftharpoons | 2 H^+ + 2e^- | 0 |
| Fe_{(s)} | \rightleftharpoons | Fe^{3+} + 3e^- | +0.04 |
| Fe_{(s)} | \rightleftharpoons | Fe^{2+} + 2e^- | +0.44 |
| Zn_{(s)} | \rightleftharpoons | Zn^{2+} + 2e^- | +0.7618 |
| Al_{(s)} | \rightleftharpoons | Al^{3+} + 3e^- | +1.662 |
It is possible, however, to “flip” the equations around. If we wanted to know the standard reduction potential for the opposite reaction of that tabulated, we could flip the reactants and products, and { E }^{\circ }_{ cell } for the reaction would be equal and opposite that of the original reaction. For example, if we wanted to know { E }^{\circ }_{ cell } for Iron III (Fe^{3+}) reducing to solid iron (Fe_{(s)}), all we have to do is write the reaction going the opposite way. In this case, Fe^{3+} + 3e^- =Fe_{(s)} would have { E }^{\circ }_{ cell }= -0.04.
Voltaic Cells – Essential Knowledge 3.C.3
Electrochemical reactions that are spontaneous can be used to generate an electric current. Voltaic (or galvanic) cells host each of the redox half reactions in different containers called half-cells. The half-cells are formed by including a metal in two different oxidation states, usually a solid electrode and an aqueous metal ion. The electrons generated from a spontaneous oxidation reaction, such as zinc metal to zinc(II) ions, are coupled to a favorable reduction reaction, like the solidifying of copper(II) ions into copper metal.
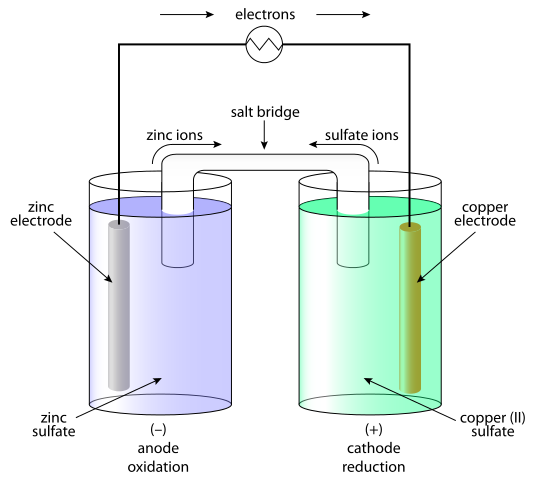
The electrodes in each half-cell are connected to allow electron flow. As electrons flow from the oxidized to the reduced metal, positive charge accumulates at the oxidized electrode and negative charge at the reduced one. To balance this accumulated charge, positive and negative ions flow into the cells from a salt bridge that connects the two half-reactions. A salt bridge is essentially an aqueous circuit filled with both positive and negative ions, which can flow freely from into each cell, and balance the accumulated charge.
Things to keep in mind:
– Electrons move from the anode to the cathode.
– The overall voltage in a voltaic cell is negative, opposite the flow of e^-.
Corrosion – Essential Knowledge 3.C.3
The last topic we’re going to consider before moving on to free response mastery is corrosion. Corrosion is a type of electrochemical reaction where pure metal reacts with and is oxidized by oxygen and water to form metal complexes which are brittle. The atom with the smaller (more negative) { E }^{\circ }_{ reduction } will be oxidized in this reaction. An example of this is the rusting of iron, which is the reaction we have been using as an example thus far. Metals can be protected from corrosion by coating them with another metal which is a more potent reducing agent. Another term for this metal is a sacrificial anode since the coating is oxidized to protect the underlying vulnerability. Many times, the sacrificial metal is zinc because it is a strong reducing agent (very likely to lose electrons for stability).
And with that, we’re done! The topic of electrochemistry sure isn’t an easy one. Many bits and pieces have to be digested, and you can’t memorize everything for your exam. So remember what we’ve taught you: use the freebies you are given (e.g., periodic table, equation sheet) to help you assign oxidation states and predict reactions based on free energy changes. And above all, take a deep breath and go for it!
Looking for AP® Chemistry practice?
Kickstart your AP® Chemistry prep with Albert. Start your AP® exam prep today.








